This is an archive copy of the IUCr web site dating from 2008. For current content please visit https://www.iucr.org.

1. Rotation Matrices and Translation Vectors

Rotation matrices (R) and translation vectors (t) are very powerful descriptions of the symmetry within the crystal and give aid in origin specification, in determining phase restrictions, systematic absences, systematic enhancement and seminvariants, in distinguishing centric and acentric reflections, general and spatial reflections and are helpful in making correct space group determinations.

Every space group has a number of equivalent positions. These may be from 1, as in P1, to 192, as in Fm3m, Fm3c, Fd3m, Fd3m and Fd3c. Every atom at a point (x, y, z) is also found as a result of the symmetry at position ($x^{\prime}, y^{\prime}, z^{\prime}$). The equivalent positions are listed for all space groups in International Tables for X-ray Crystallography . The equivalent positions are related to each other through symmetry operations. Every symmetry operation is a pair of R and t. One equivalent position is derived from another through a rotation and a translation applied in that order. The word rotation stands not only for 2-, 3-, 4- or 6-fold rotation, but also for reflections in a point or in a plane. The translations are along axes or diagonals of the unit cell. The relation between two equivalent positions can be denoted as:

$$\textbf{R} \cdot \left(\begin{array} {c} x\\ y\\ z\end{array... ...\textbf{R} \cdot \textbf{x} + \textbf{t} = \textbf{x}^{\prime}.$$ (1)

Example :

The space group P1 has only one equivalent position (x, y, z). The only symmetry operation in that space group is thus the unit matrix, I

$$\textbf{I} = \left(\begin{array} {ccc}1 & 0 & 0\\ 0 & 1 & 0\... ...textbf{t} = \left(\begin{array} {c}0\\ 0\\ 0\end{array}\right).$$

Example :

The space group P3₁ has 3 equivalent positions: (x, y, z), (-y, x - y, $\frac{1}{3}$ + z) and (y - x, -x, $\frac{2}{3}$ + z). The symmetry operations are:

$$\textbf{R}_1 = \left(\begin{array} {ccc}1 & 0 & 0\\ 0 & 1 & ... ...= \left(\begin{array} {c}0\\ 0\\ \frac{1}{3}\end{array}\right),$$

$$\textbf{R}_3 = \left(\begin{array} {ccc}-1 & 1 & 0\\ -1 & 0 ... ...= \left(\begin{array} {c}0\\ 0\\ \frac{2}{3}\end{array}\right).$$

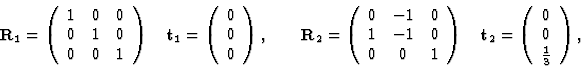
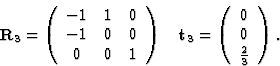
Note that

$$\textbf{R}_2 \cdot \textbf{R}_2 = \textbf{R}_3, \textbf{t}_2... ... = \textbf{R}_3 \cdot \textbf{R}_2 = \textbf{R}_1 = \textbf{I}.$$

The different symmetry operations for each space group can always be derived from at the most 3 unique not further reducible symmetry operations (3 because space is 3-dimensional). All other symmetry operations can be derived from the unique ones through:

$$\textbf{R}_i + \textbf{t}_i = \textbf{R}_m (\textbf{R}_n \{\... ...t}_m) \cdots] + \textbf{t}_0\}\ + \textbf{t}_n) + \textbf{t}_m,$$ (2)

where m, n and o can be 1, 2 or 3 for the 3 unique symmetry operations.

Copyright © 1981, 1998 International Union of Crystallography